International Journal of Pure Mathematics
E-ISSN: 2313-0571
Volume 2, 2015
Notice: As of 2014 and for the forthcoming years, the publication frequency/periodicity of NAUN Journals is adapted to the 'continuously updated' model. What this means is that instead of being separated into issues, new papers will be added on a continuous basis, allowing a more regular flow and shorter publication times. The papers will appear in reverse order, therefore the most recent one will be on top.
Submit a paper | Submission terms | Paper format
Volume 2, 2015
Title of the Paper: Spectral Equivalence of S-Decomposable Operators
Authors: Cristina Serbanescu, Ioan Bacalu
Pages: 64-69
Abstract: One of the essential characteristics of the class of decomposa-ble (spectral, scalar, generalized scalar and spectral, A-scalar) operators is the transfer of spectral proprieties from one operator to another using quasinilpotent equivalence ([14]). The family of S-decomposable operators, although larger than the class of decomposable operators studied in several papers (about 40), preserves the most interesting properties of the class of decomposable ope-rators. In this paper we make the link between S-decomposable operators and spectral equivalence (respectively, S-spectral equi-valence). As is known, for two decomposable operators T1 and T2 which are spectral equivalent, the spectral properties of T1 transfer to T2 (Theorems II.1, II.2, II.3 and Consequence II.1, [14]). We prove that this fact remains partially true for S-decomposable operators, because these operators behave differently and distinctly with respect to spectral equivalence; in this case, the spectral equivalence is not ”equivalent” to equality of spectral maximal spaces XT1 (F) = XT2 (F); this equality involves only a weaker property called S-spectral equivalence, which is natural in this case. To show the relevance and the necessity of studying the above stated property for the family of S-decomposable operators, we emphasize the consistency of this class, in the sense of how many and varied are the subfamilies that compose it: the restrictions and the quotients (with respect to an invariant subspace) of decomposable (unitary, self-adjoint, normal, spectral (scalar), genera-lized spectral (scalar), A-scalar, A-unitary) operators; the perturbations and the direct sums composed by one decomposable operator and another operator; the subscalar (subnormal, subdecomposable) operators are S-decomposable (practically, S-scalar, S-normal), as restrictions of scalar (respectively, normal, decomposable) operators. Putinar showed that the hiponormal ope-rators are subscalar, hence S-decomposable. The quasinormal operators (i.e. T commutes with T T), being subnormal, are S-decomposable; for cosubnormal operators (i.e. T is subnormal), the adjointable operators T are S-decomposable. Cesaro operators are subscalar, hence S-scalar and S-decomposable; the operators which admit scalar dilatations (extensions) (C. Ionescu-Tulcea) or A-scalar dilatations (El. Stroescu) are S- decomposable. In fact, Albrecht and Eschmeier showed that any operator is the quotient of a restriction or the restriction of a quotient of decomposable operators ([3]), thus any operator is S-decomposable or similar to an S-decomposable operator.
Title of the Paper: Estimating Parametric Derivatives of First Exit Times of Diffusions by Approximation of Wiener Processes
Authors: Sergey A. Gusev
Pages: 55-63
Abstract: The problem of obtaining estimates of derivatives with respect to parameters of mathematical expectations of functionals of diffusion processes with absorbing boundary is considered in the paper. The problem demands to obtain the parametric derivatives of first exit times for the random processes. These derivatives can be obtained from the differentiation of the equation which is the result of applying the Ito’s formula to some function that vanishes on the boundary. The problem of differentiating the Ito integral, that arises here, is solved by approximating the Wiener process by a Gaussian one with exponential correlation function, consistent with the step length of the Euler method.
Title of the Paper: Birkhoff Weak Integrability of Multifunctions
Authors: Anca Croitoru, Alina Gavriluţ, Alina Iosif
Pages: 47-54
Abstract: We define and study Birkhoff weak integrability of multifunctions (taking values in the family of nonempty subsets of a real Banach space) relative to a non-negative set function. We obtain some classic integral properties. Results regarding the continuity properties of the set-valued integral are also presented.
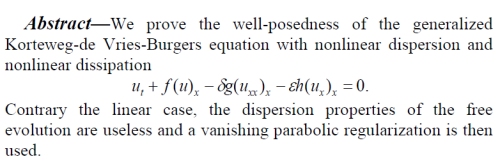
Title of the Paper: Well-Posedness of the Generalized Korteweg-De Vries-Burgers Equation with Nonlinear Dispersion and Nonlinear Dissipation
Authors: N. Bedjaoui, J. M. C. Correia, Y. Mammeri
Pages: 38-46
Title of the Paper: Operational Techniques for Laguerre and Legendre Polynomials
Authors: Clemente Cesarano, Dario Assante, A. R. El Dhaba
Pages: 30-37
Abstract: By starting from the concepts and the related formalism of the Monomiality Principle, we exploit methods of operational nature to describe different families of Laguerre polynomials, ordinary and generalized, and to introduce the Legendre polynomials through a special class of Laguerre polynomials themselves. Many of the identities presented, involving families of different polynomials were derived by using the structure of the operators who satisfy the rules of a Weyl group. In this paper, we first present the Laguerre and Legendre polynomials, and their generalizations, from an operational point of view, we discuss some operational identities and further we derive some interesting relations involving an exotic class of orthogonal polynomials in the description of Legendre polynomials.
Title of the Paper: The Relativistic Burgers Equation on a de Sitter Spacetime. Derivation and Finite Volume Approximation
Authors: Baver Okutmustur, Tuba Ceylan
Pages: 20-29
Abstract: The inviscid Burgers equation is one of the simplest nonlinear hyperbolic conservation law which provides a variety examples for many topics in nonlinear partial differential equations such as wave propagation, shocks and perturbation, and it can easily be derived by the Euler equations of compressible fluids by imposing zero pressure in the given system. In recent years, several versions of the relativistic Burgers equations have been derived on different spacetime geometries by the help of the Lorentz invariance property and the Euler system of relativistic compressible fluid flows with zero pressure on different backgrounds. The relativistic Burgers equation on the Minkowski (flat) and Schwarzshild spacetimes are obtained in [13] where the finite volume approximations and numerical calculations of the given models are presented in detail. On the other hand a similar work on the Friedmann–Lema??tre–Robertson–Walker (FLRW) geometry is described in [5]. In this paper, we consider a family member of the FLRW spacetime so-called the de Sitter background, introduce some important features of this spacetime geometry with its metric and derive the relativistic Burgers equation on it. The Euler system of equations on the de Sitter spacetime can be found by a known process by using the Christoffel symbols and tensors for perfect fluids. We applied the usual techniques used for the chwarzshild and FLRW spacetimes in order to derive the relativistic Burgers equation from the vanishing pressure Euler system on the de Sitter background. We observed that the model admits static solutions. In the final part, we examined several numerical illustrations of the given mode through a finite volume approximation on curved spacetimes based on [1, 16]. The effect of the cosmological constant is also numerically analysed in this part. Furthermore, a comparison of the static solution with the Lax Friedrichs scheme are implemented so that the results demonstrate the efficiency and robustness of the finite volume scheme for the derived model.
Title of the Paper: Investigation of (m,2)-Methods for Solving Stiff Problems
Authors: Eugeny A. Novikov
Pages: 14-19
Abstract: A family of (m,2)-methods for stiff problems solving is studied. Numerical schemes of the second and the third order are constructed. It is shown, that a maximum order of (m,2)-methods is four. A-stable and L-stable numerical formulas of a maximum order are designed. An inequality for accuracy control for the L-stable methods of the fourth order is constructed. Numerical results confirming the efficiency of the constructed method are given.
Title of the Paper: Schwartz-Christoffel Transformation Applied to Polygons and Airfoils
Authors: Etsuo Morishita
Pages: 1-13
Abstract: Potential flows around polygons and airfoils are obtained by the Schwartz-Christoffel transformation. Although the Schwartz-Christoffel transformation is well known, the application to the flow problems is limited to relatively simple flows. The present author extended the method to flows around regular and other simple polygons. This is possible by mapping a circle to a polygon. It is reminded that a flat plate from the Joukowsky transformation is also included in the Schwartz-Christoffel one. It is interesting to note that the Schwartz-Christoffel transformation can be applicable to a two-dimensional airfoil approximated as a polygon.



