International Journal of Pure Mathematics
E-ISSN: 2313-0571
Volume 5, 2018
Notice: As of 2014 and for the forthcoming years, the publication frequency/periodicity of NAUN Journals is adapted to the 'continuously updated' model. What this means is that instead of being separated into issues, new papers will be added on a continuous basis, allowing a more regular flow and shorter publication times. The papers will appear in reverse order, therefore the most recent one will be on top.
Submit a paper | Submission terms | Paper format
Volume 5, 2018
Title of the Paper: Coupled Fixed Point Theorems in Ordered Non-Archimedean Intuitionistic Fuzzy Metric Space Using K-Monotone Property
Authors: Akhilesh Jain, R. S. Chandel, Kamal Badhwa, Rajesh Tokse
Pages: 50-54
Abstract: In this paper we define k-monotone property and proved the coupled fixed point theorem in ordered non-Archimedean Intuitionistic fuzzy metric space. Our result is an extension of the results of Mohinta S., Samanta T.K.
Title of the Paper: F(x; y) ⨁ z = F(x ⨁ z; y ⨁ z)
Authors: D. Vivona, M. Divari
Pages: 44-49
Abstract: This research belongs to the field of functional equations started by J.Aczel in 1969. The aim of this paper is to present a generalization of the functional equation F(x; y) + z = F(x + z; y + z) on the pseudo-analysis. If the function F is unknown, the mentioned expression is called distributivity equation and many authors have given the solutions. Here, in order to generalize this equation, we introduce the pseudo-operations: called pseudo-addition ⨁, pseudo-difference ⊖ and pseudo-multiplication ⊙ Then, we transform the mentioned equation in another equation in which the common addition + has been replaced by the pseudo-addition ⨁: We study the new equation: F(x; y) ⨁ z = F(x ⨁ z; y ⨁ z) and we are able to find the solutions. Really, we give the class of all solutions, depending on two arbitrary functions.
Title of the Paper: Filters in Michálek's Fuzzy Topological Spaces
Authors: Francisco Gallego Lupiáñez
Pages: 41-43
Abstract: The aim of this paper is to study some properties of filters in Michálek's fuzzy topological spaces, which are quite different of the classic properties of fuzzy topology. That continues a previous paper of this author.
Title of the Paper: On New Orthogonal Contractions in b-Metric Spaces
Authors: O. Yamaod, W. Sintunavarat
Pages: 37-40
Abstract: In this paper, we introduce the concept of an s-orthogonal contraction in the sense of b-metric spaces by using the notion of the orthogonal sets. We also establish some fixed point theorem for the purposed contraction and state some illustrative example to claim that our results properly generalize some results in the literature. Further, by using the main results, we prove some fixed point results for orthogonal contraction in metric spaces. Our results generalize and improve the result of Gordji et al. [3] and several well-known results given by some authors in metric and b-metric spaces.
Title of the Paper: A Generalized Hardy-Rogers Type with φ-Best Proximity Point Result
Authors: Aphinat Ninsri, Wutiphol Sintunavarat
Pages: 33-36
Abstract: The concept of a Hardy-Roger contraction selfmapping and its fixed point results have many applications in the various branches in mathematics. However, these results can not be applied in the global optimization problems. This is the motivation for improving the idea of a Hardy-Rogers contraction self-mapping to the sense of nonself-mappings since the best proximity point result for such mappings has some relation with the global optimization problems. The main aim of this paper is to define the new generalized Hardy-Rogers nonself-contraction mappings and prove the best proximity point result for such mappings.
Title of the Paper: Coupled FCT-HP for Analytical Solutions of the Generalized Time-Fractional Newell-Whitehead-Segel Equation
Authors: S. O. Edeki, J. I. Ejiogu, S. A. Ejoh, G. A. Adeyemi
Pages: 29-32
Abstract: This paper considers the generalized form of the time-fractional Newell-Whitehead-Segel model (TFNWSM) with regard to exact solutions via the application of Fractional Complex Transform (FCT) coupled with He’s polynomials method of solution. This is applied to two forms of the TFNWSM viz: linear and nonlinear versions of the time-fractional Newell-Whitehead-Segel equation whose derivatives are based on Jumarie’s sense. The results guarantee the reliability and efficiency of the proposed method with less computation time while still maintaining high level of accuracy.
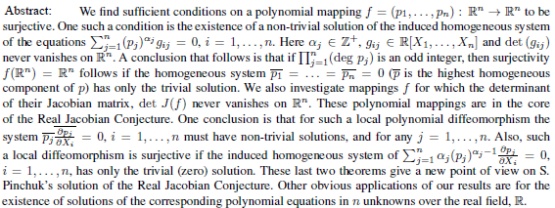
Title of the Paper: Fibers of Polynomial Mappings Over Rn
Authors: Ronen Peretz
Pages: 24-28
Title of the Paper: Analytical Solutions of a 1D Time-fractional Coupled Burger Equation via Fractional Complex Transform
Authors: S. O. Edeki, G. O. Akinlabi
Pages: 19-23
Abstract: In this paper, we obtain analytical solutions of a system of time-fractional coupled Burger equation of one-dimensional form via the application of Fractional Complex Transform (FCT) coupled with a modified differential transform method (MDTM). The associated fractional derivatives are in terms of Jumarie’s sense. Illustrative cases are considered in clarifying the effectiveness of the proposed technique. The method requires minimal knowledge of fractional calculus. Neither linearization nor discretization is involved. The results are also presented graphically for proper illustration and efficiency is ascertained. Hence, the recommendation of the method for linear and nonlinear space-fractional models.
Title of the Paper: Fuzzy Semi – Alpha – Compactness and Fuzzy Semi – Alpha – Closed Spaces in Fuzzy Topological Spaces
Authors: Raja Mohammad Latif
Pages: 6-18
Abstract: This research paper deals with the concept of fuzzy semi – alpha compact space as well as fuzzy semi alpha – closed space setting of a fuzzy topological space. We also investigate the relationships between fuzzy semi alpha – almost compactness and fuzzy semi alpha – nearly compactness. We present a number of properties and characterizations of these notions of fuzzy semi – alpha compact space, fuzzy semi alpha – closed space, fuzzy semi alpha – almost compact space and fuzzy semi alpha – nearly compactness in fuzzy topological spaces.
Title of the Paper: One Approach for Aggregation of Fuzzy Estimates of Several Groups of Experts
Authors: Teimuraz Tsabadze, Irakli Chelidze
Pages: 1-5
Abstract: This paper introduces one approach to group decision-making, where experts’ opinions are expressed by fuzzy sets. It is meant that there are several groups of experts and, therefore, several finite collections of fuzzy sets turn out. Then a quite simple approach for aggregation of obtained finite collections of fuzzy sets into resulting one is proposed and its algorithm is determined.



